This page is part of a series on insolation-factors.
An overview is provided at insolation.
The pages are:
sun angle,
air mass,
day length, and
clouds and pollution.

As discussed on sa&i intro, the intensity of the solar radiation striking a horizontal surface is lower when the sun is lower in the sky.
On this page we present a table that shows how much insolation falls on a horizontal surface when the sun has a given "solar elevation angle" (aka: "SEA" - its the angle of the sun above the horizon).
This page also attempts to interpret the data and relate it to solar energy collection in different parts of the world.
An explanation of the precise trigonometric relationship between solar elevation angle and the insolation
on a horizontal surface is at
sa&i formula.
On sa&i intro, we use a diagram similar to the one below this paragraph to explain that when the sun is lower in the sky, a square meter of its rays are spread out over more than a square meter of the ground, reducing their concentration and thus their strength.
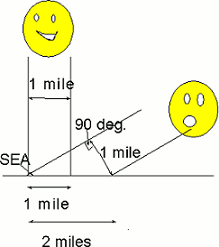
On that page we also point out that tilting our solar panels so that the sunlight is hitting them directly can keep the "spreading-out" effect from impacting them.
Before we jump into the table, lets explain why the table takes
air mass
into account but not day length.
You'll recall from sa&i intro that both air mass and day-length are influenced by the solar elevation angle and that both air mass and day-length influence how much solar radiation a place receives.
So, why not put them both in a table about the relationship between sun angle and insolation?
Both the "spreading-out" of sunlight discussed in sa&i intro and air mass reduce the amount of solar radiation a place receives each moment. As we mention in the sa&i intro, we can compensate for the "spreading-out" problem by tilting our solar collectors so that the sun hits them more directly but we can't do anything about the effect of air mass.

If, for example, the sun is 30 degrees above the horizon, the resulting air mass lowers the sun's intensity by almost 20% before it even reaches our solar panels. If we ignored the effects of air mass, we would paint an overly-optimistic picture of the solar radiation on a horizontal surface.
Therefore, to get a feel for how much the sun angle really affects the amount of insolation striking a horizontal surface each moment, it is useful to start with how intense the solar radiation is after the air mass has reduced it.
We can then reduce that already-reduced solar intensity with the "spreading-out" effect of the sun angle we're studying.
An examples of this process in action is given in our interpretation of the table.
Since day-length doesn't impact the moment-to-moment solar radiation a place receives (it impacts how much solar radiation a place receives over the course of the day but not in a given moment), we didn't include day-length in this table.
The table only shows how the solar elevation angle affects the amount of solar radiation striking a horizontal surface in a given moment. [For a discussion of the difference between instantaneous and long-term insolation see insolation definitions.]
"And now, without further ado, the table!"
| SEA° | W/m2Max. W/m2 after AM | % of Max. W/m2 on HS | W/m2 on HS |
| 90° | 1040 | 100% | 1040 |
| 75° | 1030 | 97% | 1000 |
| 60° | 1000 | 87% | 870 |
| 45° | 950 | 71% | 675 |
| 30° | 840 | 50% | 420 |
| 20° | 710 | 34% | 240 |
| 15° | 620 | 26% | 160 |
| 10° | 470 | 17% | 80 |
| 5° | 260 | 9% | 235 |
| 0° | 20 | 0 | 0 |
The table first shows the angle of the sun above the horizon (the "solar elevation angle" or "SEA"). It then shows the intensity of the sunlight in watts per square meter (W/m2) after the air mass (AM) resulting from this sun angle has reduced the sunlight's strength. These calculations are covered on our pages on air mass.
Third, the table shows the percentage (%) of the total solar radiation in the air that will strike a horizontal surface. That is, it tells us what fraction of the solar radiation that would strike an optimally tilted solar panel will strike a solar panel laid out flat on the ground.
This is the step that tells us how large a given sun angle's "spreading-out" effect is. These numbers
are calculated with the formula from
sa&i formula.
Fourth and finally, the table shows us how much sunlight will strike a horizontal surface. It is found by converting the percentage in the third column into a decimal and multiplying that by the watts per square meter in the second column.
As an example of how this all works, take a solar elevation angle of 20.° In that case, the air mass will reduce the solar radiation in the air down to about 710 W/m2 and the sunlight will be so spread-out over a horizontal surface that it will only be about 34% as strong as it is in the air and so about 710 W/m2 * .34 = 240 W/m2 of solar radiation will strike a solar panel.
It should be noted that this table assumes clear skies. Clouds and/or pollution would lower the sunlight's intensity. Also, the air mass data assumes that you are at sea level. At higher elevations, the air mass would be less and so the sunlight's intensity would be greater.
The table gives us an idea of when we have to be extra sure to optimally tilt our solar collectors and when we aren't going to get much solar radiation no matter what we do.
I will attempt to also talk about what this data might mean to people employing solar collectors in locations along different lines of latitude. These thoughts are not at all meant to be definitive. They are just some thoughts. Anyway, people should always carefully evaluate their individual situation before deciding how to install solar panels.
| SEA° | W/m2Max. W/m2 after AM | % of Max. W/m2 on HS | W/m2 on HS |
| 90° | 1040 | 100% | 1040 |
| 75° | 1030 | 97% | 1000 |
| 60° | 1000 | 87% | 870 |
| 45° | 950 | 71% | 675 |
| 30° | 840 | 50% | 420 |
| 20° | 710 | 34% | 240 |
| 15° | 620 | 26% | 160 |
| 10° | 470 | 17% | 80 |
| 5° | 260 | 9% | 235 |
| 0° | 20 | 0 | 0 |
With the sun 90 degrees above the horizon (SEA° = 90°), the air mass lowers the intensity of the
sunlight from the 1,367 W/m2 that it is in outerspace down to about 1040 W/m2.
"The solar elevation angle is 90°" is just another way to say "the sun is directly overhead." Therefore, at this solar elevation angle, the sunlight strikes a horizontal surface directly and there is no "spreading-out" effect at all. So, the sunlight's intensity is 1040 W/m2 on a horizontal surface (column 4).
Even down to a solar elevation angle of 75°, the effects of both air mass and the "spreading-out" effect are pretty minor.
At SEA° = 60°, air mass's impact on insolation is still pretty low (1000 W/m2 is 96% of 1040 W/m2) but the "spreading-out" effect is starting to be a bigger deal (the sunlight striking a horizontal surface is now more than 10% less powerful than the sunlight striking an optimally tilted solar panel).
Therefore, starting at a solar elevation angle of about 60°, we should probably pay more attention to the tilt of our solar panels.

On the equator, the lowest the solar noon solar elevation angle ever is is around 67° (this happens on the Winter and Summer Solstices).
Of course, on a given day the sun is highest at solar noon and lower at all other parts of the day. Still, it seems that if you lived on the equator, you wouldn't do too poorly to just lay your solar panels out flat and forget about them.
When the sun is 45° above the horizon, the effect of air mass is starting to be felt although it still isn't too terrible (950 W/m2 is 91% of 1040 W/m2).
However, at this sun angle the "spreading-out" effect is a pretty big deal (the sunlight striking a horizontal surface is now almost 30% less than the sunlight striking an optimally tilted solar panel).
So, at a solar elevation angle of 45°, we really have to make sure we have a decent tilt-angle for our solar collectors.
| SEA° | W/m2Max. W/m2 after AM | % of Max. W/m2 on HS | W/m2 on HS |
| 90° | 1040 | 100% | 1040 |
| 75° | 1030 | 97% | 1000 |
| 60° | 1000 | 87% | 870 |
| 45° | 950 | 71% | 675 |
| 30° | 840 | 50% | 420 |
| 20° | 710 | 34% | 240 |
| 15° | 620 | 26% | 160 |
| 10° | 470 | 17% | 80 |
| 5° | 260 | 9% | 235 |
| 0° | 20 | 0 | 0 |
At the Tropic of Cancer (a line of latitude at about 23.3° North), the sun is directly overhead at solar noon on the Summer Solstice.
At the Tropic of Capricorn (a line of latitude at about 23.3° South), the sun is directly overhead at solar noon on the Winter Soltice (the Southern Hemisphere's seasons are the opposite of the Northern Hemisphere and the Solstices were named by people in the Northern Hemisphere).
At the spring and fall Equinoxes, the solar noon solar elevation angle is about 67° for both these lines of latitude. The lowest solar noon solar elevation angle either of these lines of latitude ever experience is about 46° (Winter Solstice for Cancer and Summer Solstice for Capricorn).
Therefore, at winter on these latitudes, you lose quite a bit of solar energy if you don't tilt your solar collectors but the rest of the year it isn't such a big deal.
When the sun is 30 degrees above the horizon, air mass reduces the intensity of the sunlight by quite a bit (840 W/m2 is 81% of 1040 W/m2), and the spreading-out effect is huge.
So, when the solar elevation angle is 30°, solar collectors can still do fairly well with the proper tilt but will do terrible if they are laid out horizontally.
At latitudes 40° North and 40° South, the highest solar noon solar elevation angle is about 73° (on the Summer Solstice for 40° North and the Winter Solstice for 40° South) and the lowest solar noon solar elevation angle is about 27° (on the WS for 40° North and the SS for 40° South). On the Equinoxes, the solar noon solar elevation angle for both of these places is about 50°.
So, at mid-latitudes, you really ought to think quite a bit about the tilt of your solar panels. You might even consider making them adjustable and adjusting the tilt each season.
It surprised me to see that you had to bring the sun down lower than 15° above the horizon before air mass halved the strength of the sun in the sky.

In London, England (51° North), the poor sun is not even quite 16 degrees above the horizon on solar noon on the Winter Solstice. At the Equinoxes, the solar noon solar elevation angle is not quite 40°.
Even on the Summer Solstice the solar noon solar elevation angle is only 62 degrees above the horizon. And I haven't even mentioned the clouds!
Well, if a Londoners invests in solar panels he or she shouldn't expect to get too much out of them in December. Also, definitely never just lay them down flat on the ground and hope for the best.
The formula that relates sun angle and insolation is at sa&i formula; sa&i intro introduces the concepts quantified on this page. We also have several pages on tilt angles.
If this page has been a help to you
please recommend it with Google+ below:
Updated: 02/18/2015