This page is part of a series on insolation-factors.
An overview is provided at insolation.
The pages are:
sun angle,
air mass,
day length, and
clouds and pollution.
On this page we explain the precise mathematical relationship between the angle of the sun in the sky and the portion of the available insolation that will strike a horizontal surface. We also explain the formula that calculates the optimal solar collector tilt angle for a given moment.
Our plan of attack for this page is to first give the formulas and show how to use them and then demonstrate why they're true.
An introduction to the concepts on this page (and more!) is at sa&i intro. Examples relating various sun angles to the corresponding insolation levels (and more!) is at sa&i data.
1. Sun Angle and Insolation:
[sin(SEA°) = (portion of the available sunlight that will fall on a horizontal surface)], where "SEA" = "solar
elevation angle" which is the angle of the sun above the horizon.
For example, if the sun is 30 degrees above the horizon, we have sin(30°) = .5 and so the sunlight on a horizontal surface will be half as intense as the sunlight in the air. So, if due to say a few clouds and the effects of air mass, the strength of the sunlight in the air was about 700 W/m2, 350 W/m2 would strike a horizontal surface.
2. Optimal Tilt Angle for a given moment:
[Optimal Tilt Angle = 90° - SEA°].
For example, if on a given moment, the sun was 40 degrees above the horizon, at that moment the optimal tilt angle would be 90° - 40° = 50°.
We will now explain why these two equations are true.
In all that follows, we will be talking about "surface area" (a two-dimensional quantity) but often mixing up "surface area" with "surface lengths" (a one-dimenstional quantity). We are allowed to do this because we are assuming the unmentioned dimension is always the same. [This means that the unnamed dimension always cancels out: ((L1 * L3)/(L2 * L3)) = (L1/L2).]
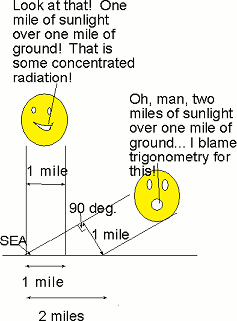
As we discus in more detail on sa&i intro, the problem is that the lower the sun is in the sky, the more "spread-out" the sunlight falling on a horizontal surface is.
That means that the sunlight falling on a horizontal surface is less concentrated than the sunlight in the air and so if we just lay our solar panels out flat on the ground, they will miss out on a lot of solar radiation.
We want to be able to use the angle of the sun above the horzion (SEA°) to solve for:
(portion of the available sunlight that will fall on a horizontal surface)
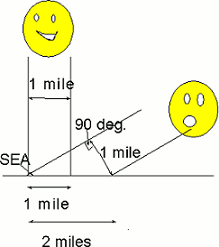
First use the color-coded diagram below to see that the intensity of the sunlight striking an optimally tilted solar collector (one that is tilted so that the sunlight strikes it perpendicularly and thus isn't spread-out at all) is equal to the intensity of the sunlight in the sky. In both cases the sunlight is as concentrated as possible.
Therefore (as the following example will help clarify), we can learn how intense the sunlight striking a horizontal surface is in relationship to how strong it is in the air by comparing the surface area covered by a sunbeam of sunlight falling on a horizontal surface with the surface area that the same sunbeam would cover on an optimally tilted surface.
If, for example, you need a three meter horizontal solar panel to collect the same amount of solar radiation as you can collect with one meter of an optimally tilted solar panel, it must be that per area the solar radiation falling on the horizontally tilted solar panels is 1/3 as concentrated as the solar radiation falling on the optimally tilted solar panel.
Therefore, the intensity of the solar radiation falling on a horizontal surface will be 1/3 the intensity of the sunlight in the air.
Mathematically,
[(area of optimally tilted surface)/(area of horizontal surface covered by same amount of sunlight)]
= (portion of the available sunlight that will fall on a horizontal surface)
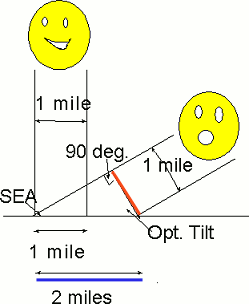
The diagram is color-coded to match our explanation.
We draw a right triangle with the hypoteneuse equal to the
(length of the horizontal surface covered by the sunbeam).
We then see that the line across from the angle of the sun above the horizon (SEA) is the trigonometric "side opposite". Furthermore, the length of this line is equal to the length of an optimally tilted solar collector being hit by the same sunbeam.
Therefore, [(side opposite)/(hypoteneuse)] is equal to [(length of optimally tilted surface)/ (length of horizontal surface covered by same amount of sunlight)]. This, however, is equal to what we are trying to discover, namely: (portion of the available sunlight that will fall on a horizontal surface).
But according to
trigonometry,
sine = (side opposite)/(hypoteneuse).
And so,
sin(SEA°) = [(length of optimally tilted surface)/
(length of horizontal surface covered by same amount of sunlight)] =
(portion of the available sunlight that will fall on a horizontal surface).
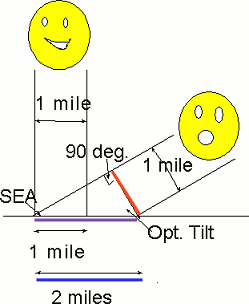
The optimal tilt for a given moment, is the tilt that keeps the sunlight on the surface of the solar collector as intense as the sunlight in the air at that moment. It is the angle that keeps the sunlight on the surface as concentrated (or "un-spread-out") as the sunlight in the air. As you can see from the diagram, this angle is also part of the right triangle we just drew.
It is the only "unknown" angle in our right triangle. In a right triangle, one angle will of course always be 90° and in our right triangle one angle is SEA° and so it is easy to find the optimal tilt. It must equal (180° - 90° - SEA°) = (90° - SEA°).
We have several pages on tilt angles.
The optimum tilt for a given day is the tilt that factors in all the different optimum tilts for a given moment and comes up with the tilt that makes the most sunlight hit the solar panel as directly as possible for as much of the day as possible. The optimum tilt for a given month would factor in all the days in that month. To find the optimum tilt angle for a year you'd factor in all the days of the year.
However, a tilt angle that is designed solely to compensate for the "spreading-out" effect of lower sun angles fails to account for many other factors such as clouds, diffuse radiation and air mass.
As we discuss in sa&i intro, since diffuse radiation is distributed evenly throughout the sky, the steeper your tilt, the more diffuse radiation you miss out on and so you gather the most diffuse radiation with a horizontal solar panel. Diffuse radiation accounts for about half of the radiation in many high latitude, cloudy places like Northern Europe.
Air Mass is a measure of how much atmosphere the sunlight has to pass through on its way to the sky and the lower the sun is in the sky, the more the effect of "air mass" reduces how much insolation makes it to the surface of the earth. Unlike clouds and diffuse radiation, "air mass" is directly related to the angle of the sun in the sky and so it can be calculated in the general case (without factoring in local weather patterns). [More on tilt angles.]
Air mass's effect on insolation is often significant. It is important to factor in the effects of air mass if you want to get a realistic idea of how much solar radiation will fall on a horizontal surface with a given sun angle under clear skies.
This is explained and many examples of how insolation on a horiztontal surface varies with sun angle is all at sa&i data.
If this page has been a help to you
please recommend it with Google+ below:
Updated 02/18/2015