This page is part of a series on insolation-factors.
An overview is provided at insolation.
The pages are:
sun angle,
air mass,
day length, and
clouds and pollution.
As discussed in air mass intro, "air mass" measures how much atmosphere sunlight has to pass through.
Since atmosphere absorbs and scatters solar radiation, a larger air mass means less insolation. When the sun is lower in the sky, the sunlight has to pass through more atmosphere and so the air mass is greater.

On this page, we explain the mathematical formula that calculates how air mass varies with sun angle.
The plan is to develop an approximation for the relationship between air mass and sun angle.
We will then give the more precise formula and show off how close the
approximation was using a table of air mass and sun angle data stolen from
air mass intro.
The approximation to be developed is: Air Mass = 1/cos(ZA°) where "ZA" stands for "zenith angle" which is how far away from directly overhead the sun is. We will discuss "zenith angle" more on this page and a more thorough treatment of angles is at: insolation definitions.
Please note: both this approximation and the precise formula given later ignore the effects that altitude has on air mass and so they are to be used only at sea level.
Take a quick look at the diagram below. Does it already
makes sense to you that since "air mass" measures how many times more atmosphere
the sunlight has to pass through than it would have to pass through if the sun was directly
overhead, air mass = Y/X? If so, you should
skip to the trigonometry
right now. If you choose to read the more thorough introduction of these concepts, never forget that at any moment you can cut to the
chase and scroll down to the trigonometry!
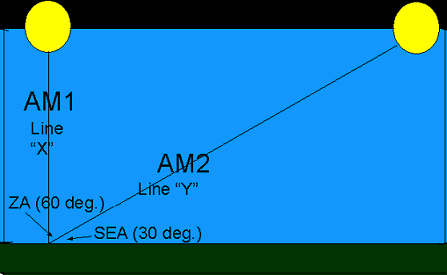

On air mass intro, we explain that a given moment's "air mass" is expressed as a ratio between how much atmosphere the sunlight has to pass through right that moment and how much atmosphere the sunlight would have to pass through if the sun was directly overhead.
To approximate the air mass, we imagine that things are as they appear to us when we stand outside: the horizon is perfectly flat and the atmosphere is a flat layer of air above it.
We then draw two lines from the surface of the earth to two different points at the top of the atmosphere.

In the diagram above, the blue space is the atmosphere and the black space is outer space. The dark green space is the ground.
The yellow circle at the top of line 'X' is where the sun's rays would've entered the atmosphere if the sun was directly overhead. Regardless of where the sun really is in the sky, "Line 'X'" always represents how far the sun's rays would have to travel through the atmosphere if the sun was directly overhead.
Let's suppose that the sun at the end of line 'Y' is where the sun really is in the sky at a given moment. Then "Line 'Y'" is how far the sun's rays actually do have to travel through the atmosphere at that moment.
Since "air mass" is defined as how many times more atmosphere the sunlight has to cross than it would've had to cross if the sun was directly overhead, it answers the question, "how many times bigger is 'Y' than 'X'?". Another way to say this is "air mass = 'Y/X'".

The smaller the angle of the sun above the horizon ("SEA" = "solar elevation angle") is, the longer 'Y' is. 'X', on the other hand, always has the same length.
So, the lower the sun is in the sky, the bigger 'Y' is in comparison to 'X' and therefore the bigger 'Y/X' is. So, a lower solar elevation angle results in a larger "air mass".
In the diagram, the angle of the sun is low enough that 'Y' is twice as long as 'X'. That means its rays have to pass through twice the amount of atmosphere that they would have had to pass through if the sun was directly overhead and so we say "the air mass is 'two'" (or "AM2").
Mathematically: with 'Y' = '2X', "air mass = 'Y/X'" becomes "air mass = '2X/X' = '2'.
We get the most possible solar radiation when the sun is directly overhead. In this case, 'Y' = 'X' and the air mass is '1' ('X/X' = 1).
This relationship can be defined more precisely with trigonometry.
[For those just joining us: Line 'X' represents how far the sun's rays would have to travel through the atmosphere if the sun was directly overhead. Line 'Y' is how far the sun's rays actually do have to travel through the atmosphere.]
If we know the angle of the sun in the sky, we can use a trigonometric relationship to find out the ratio between 'X' and 'Y'. To see this let's replay that last diagram. In the diagram below, 'ZA' stands for the zenith angle.
As I said in the intro, "zenith angle" is how many degrees away from directly overhead the sun is. Mathematically, the relationship between the "zenith angle" and the angle of the sun above the horizon (the "solar elevation angle") is [ZA° = (90° - SEA°)] and [SEA° = (90° - ZA°)]. If the sun is directly overhead it is 90 degrees above the horizon and SEA° = 90°, making ZA° = 0°. [more: insolation-definitions]

Trigonometry informs us that the triangle in the above diagram is a right triangle with line 'Y' as the hypoteneuse and line 'X' as the line that is "adjacent" to ZA.
Trigonometry would also like to point out that in a right triangle, the secant of an angle is equal to the hypoteneuse divided by the line adjacent to that angle. That is, [secant = (h/a)]. We then have: sec(ZA°) = Y/X.
Since (secant = (1/cosine)) and people often feel more comfortable hanging out with cosines than they do hanging out with secants, this relationship is often stated as: (1/cos(ZA°)) = Y/X. But we've defined "air mass" as 'Y/X' and so [air mass = (1/cos(ZA°))].

An example: when the center of the sun is 30° above the horizon, the zenith angle is 90° - 30° = 60°
[(1/cos(60°)) = 2]. So, when
the sun is 30° above the horizon, the air mass is 2.
Another example: when the center of the sun is on the horizon, it is 0°
above the horizon, the zenith angle is
90° - 0° = 90.° [(1/cos(90°)) = 1/0] which is undefined and so...um...
Wait! Let's try it with secant. Then we get sec(90°) = infiniti. So, there, that's much better. When the center of the sun is on the horizon, its rays have to travel an infinite distance to reach us! Oh, no, that doesn't sound so great either. Hmmm...
Silly us! We forgot that we were just finding a way to estimate the air mass! To approximate the air mass, we ignored the curvature of the earth.
They have made a formula that corrects for the curvature of the earth and is thus more precise. It goes like this: AM = 1/[cos(ZA°) + (.50572 * (96.07995 - ZA°)-1.6364)] (Source: 2).
The closer to directly overhead the sun is, the less the curvature of the earth affects how much atmosphere the sunlight has to go through. This means that the precise formula varies from the estimation more when the sun is lower in the sky.
| SEA | Est. | Air Mass | W/m2 | ||
| 90° | 1.00 | 1.00 | 1040 | ||
| 75° | 1.04 | 1.03 | 1030 | ||
| 60° | 1.15 | 1.15 | 1000 | ||
| 45° | 1.41 | 1.41 | 950 | ||
| 30° | 2.00 | 1.99 | 840 | ||
| 20° | 2.92 | 2.90 | 710 | ||
| 15° | 3.86 | 3.81 | 620 | ||
| 10° | 5.76 | 5.59 | 470 | ||
| 5° | 11.5 | 10.3 | 260 | ||
| 1° | 57.3 | 26.3 | 56 | ||
| 0° | - | 38 | 20 | ||
| * The air mass formula was not as precise for SEA° = 0° so I only rounded to the nearest whole number; The formulas for this table are here: 1. | |||||
To see this, look at this table which compares the estimated air mass (Est.)
to the actual air mass Air Mass) for various solar elevation angles (SEA - the angle of the sun above the
horizon).
Unless the sun is extremely low in the sky, the approximate formula works very well. Even when the sun is 30° above the horizon (SEA° = 30°; ZA° = 60°), there is no difference between the air mass value we got rounding up from the air mass calculated with the precise formula and the air mass value we estimated with 1/cos(ZA°).
The imprecision doesn't really become particularly pronounced until the sun is 5° above the horizon (ZA° = 85°). Then the approximation is about 10% too high (AM11.5 instead of the correct value of AM10.3) When the sun is on the horizon (SEA° = 0°; ZA° = 90°), the approximation is almost twice as high as the real air mass.
Since the larger the air mass value, the more the insolation is reduced (see air mass intro), the approximation overestimates how much insolation will be lost to air mass at a given sun angle.
[The numbers on this chart assume clear skies and that the surfaces of the solar collectors are at sea level and are angled perpendicular to the sun's rays. The W/m2 represents the total global radiation resulting from the corresponding real air mass.]
The introduction of air mass (air mass intro) includes a table that shows the impact of altitude above sea level on air mass and insolation. Our pages on sun angle and insolation also discuss air mass. One of those pages - sa&i data shows how air mass impacts the amount of solar radiation falling on a horizontal surface.
If this page has been a help to you
please recommend it:
1. The calculations for this table were made using formulas from pveducation.org (http://www.pveducation.org/).
The page is pved. air mass. The formula
determining the relationship between solar angle and air mass is the one that I give in the section:
Precise vs Approximate Formulas. The relationship
between air mass and insolation is found using: IG = 1.1*ID and ID = 1.353*(0.7)(AM * .678) where
IG stands for the total global radiation (this is the number I used in the table) and ID stands for the intensity of
the direct (as opposed to diffuse) light.
2. This formula came from the pveducation page listed in footnote #1.