This page is part of a series on insolation-factors.
An overview is provided at insolation.
The pages are:
sun angle,
air mass,
day length, and
clouds and pollution.
Air mass is a measure of how much atmosphere the sun's rays have to pass through on their way to the surface of the earth. Since particles in the atmosphere absorb and scatter light rays, the more atmosphere solar radiation passes through on its way to us, the less solar energy we can expect to get.

This page defines "air mass" (as a ratio) and explains and quantifies how sun angle determines air mass and how air mass in turn determines insolation.
We also examine the impact of air mass in different parts of the world.
An explanation of the mathematical relationship between air mass and solar angle as well as a look at the accuracy of the most common air mass formula [AM = 1/cos(ZA°)] is at this page: air mass formula.
As we said above, "air mass" (abbreviated "AM") measures how much atmosphere sunlight has to pass through.
The air mass at any given moment is expressed as a ratio between how much atmosphere the sunlight has to pass through right that moment and how much atmosphere the sunlight would have to pass through if the sun was directly overhead.
It isn't that there is ever more than one atmosphere over our heads, it is just that sometimes the sunlight has to travel though more of it than at other times and so the effect is as if the sunlight had to travel through more than one atmosphere.

When the sunlight is directly overhead (90 degrees above the horizon), the sunlight has to go through the least possible amount of atmosphere and we say that "the air mass is 1" (AM1). Any air mass greater than "1" tells us how many times more atmosphere the sunlight has to travel through than it would have to travel through if the sun was directly overhead.
For example, an air mass of "2" (AM2) means that the sunlight has to go through twice as much atmosphere as it does when the air mass is "1" (AM1). As we will see, lower sun angles make for greater air mass values.
The closer the sun is to the horizon, the longer its path across the sky is and the larger the air mass is. This can be seen from the diagram below.
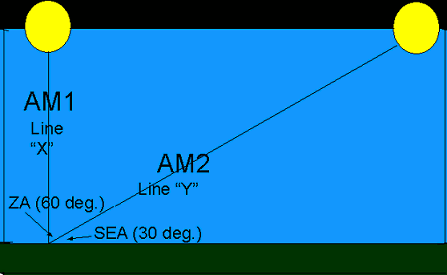
"AM1" is the air mass when the sun is directly overhead and the least amount of atmosphere needs to be crossed to reach the earth's surface.
When the sun is 30 degrees above the horizon (SEA° = 30°; SEA = "solar elevation angle"), the path that the sunlight takes through the atmosphere (line "Y") is twice as long as the path it takes when the sun is directly overhead (line "X") and so the air mass is "2" (AM2). This is the gist of why the air mass is larger when the sun is lower in the sky.
Because the sun moves through the sky during the course of the day and also changes its path throughout the seasons, air mass is constantly changing.
We go into more detail about the mathematics behind exactly how solar angle influences air mass at air mass formula. For now we'll just give some examples of the air masses that result from various sun angles as well as how these air masses impact insolation.
Just as there is a quantifiable relationship between sun angle and air mass, there is a quantifiable relationship between air mass and the strength of the sun at a given moment.
Higher altitudes complicate things somewhat because the higher you go above sea level, the less atmosphere there is and the more the composition of that atmosphere differs from the atmosphere at sea level.
The relationship between air mass and insolation has been experimentally determined. A formula relating the two is in this footnote: 1. That footnote also houses the rest of the formulas used in the table.
| SEA | Air Mass | W/m2 | AM @ 1mi (1.6 km) | W/m2 @ 1mi (1.6 km) | |
| 90° | 1.00 | 1040 | .827 | 1090 | |
| 75° | 1.03 | 1030 | .855 | 1080 | |
| 60° | 1.15 | 1000 | .954 | 1050 | |
| 45° | 1.41 | 950 | 1.17 | 1000 | |
| 30° | 1.99 | 840 | 1.65 | 900 | |
| 20° | 2.90 | 710 | 2.40 | 780 | |
| 15° | 3.81 | 620 | 3.15 | 680 | |
| 10° | 5.59 | 470 | 4.62 | 540 | |
| 5° | 10.3 | 260 | 8.51 | 325 | |
| 1° | 26.3 | 56 | 21.8 | 83 | |
| 0° | 38 | 20 | 31 | 37 | |
| * The air mass formula was not as precise for SEA° = 0° so I only rounded to the nearest whole number; The formulas for this table are here: 1. | |||||
The first four columns of the table assumes you solar panels are at sea level. They show the relationship between how high the sun is above the horizon (SEA = solar elevation angle), the corresponding air mass (AM) and the total global radiation (in W/m2) resulting from that air mass.
The last two columns are for solar panels that are 1 mile (1.6 kilometers) above sealevel. First the air mass (AM @ ... ) is shown and then the corresponding insolation (W/m2 @ ... ).
As you can see, the effect of air mass on insolation is pretty neglible when the sun is very high in the sky and isn't even too pronounced when the sun is as low as 30° above the horizon. In fact, the air mass doesn't halve the strength of the sunlight until the sun is less than 15 degrees above the horizon.
The effect of altitude above sea level increases as the sun angle gets smaller. Our mile-high example does a good job of demonstrating this. At a solar elevation angle (SEA) of 90°, being a mile above sea level adds 5% more insolation (1090/1050 = 1.05), when the sun is 30° above the horizon, it adds 7%, SEA = 10° adds 15%, SEA = 1° adds almost 50% and SEA = 0° adds 85%.
[These numbers assume clear skies and that the surface of the solar collectors are angled perpendicular to the sun's rays (see sun angle and insolation or tilt angle for more on why you need tilt solar panels. The source for the chart and is here: 1.]
The effect of air mass is most felt when the sun is lower in the sky and so it has a bigger impact on the insolation of high latitute places. An example follows.
For the purpose of this section, keep these two places in mind as references:
(1) In London, England (latitude 51° North),
in the middle part of the day the sun is about 60° above the horizon in summer, 35° above the horizon in the spring and fall and 15°
above the horizon in winter.
(2) In Cairo, Egypt (latitude 30° North), in the middle part of the day the sun's position above the horizon is
about 80° in summer, 55° in the spring and fall and 35° winter.
As you can see from the table, even when the sun is as low as 60° above the horizon, the air mass is still quite close to "1" and the insolation level is almost at its maximum. Down to 45° above the horizon, the air mass hasn't yet doubled and the sun's intensity is over 90% of its maximum.

Even when the sun is only 30° above the horizon it still has over 80% of its power. As the sun drops below 30°, the sun's intensity starts to drop off quite a bit faster and by the time the sun is 15° overhead, it has been reduced down to 60% of its overhead strength.
From this you can see that air mass plays a very big role in the amount of sunlight London gets in the middle of the day in winter but a much lesser role in summer. Also, the effect of air mass on Cairo's noontime sun appears to be pretty much neglible in summer and not even that marked in winter.
The closer you get to the equator, the less important air mass is in determining your midday insolation levels and this is of course the time of day with the most solar radiation.
The mathematical relationship between air mass and solar angle as well as a look
at the accuracy of the most common air mass formula [AM = 1/cos(ZA°)] is at this page:
air mass formula. Our pages on
sun angle and insolation also discuss
air mass. One of those pages - sa&i data - shows
how air mass impacts the amount of solar radiation falling on a horizontal surface.
If this page has been a help to you
please share or recommend it:
Updated: 11/12/2016
1. The formulas used for this table are as follows:
1. The formula relationg solar elevation angle (SEA°) to air mass at sea level is:
Air Mass = [sin(SEA°) + (.50572 * (SEA° + 6.07995)-1.6364)](-1)
2. The air mass above sea level (AM') was estimated using this formula:
AM' = AM * (e(-.0001184 * h)) where "AM" is the sea level air mass for a given solar elevation angle,
"e" stands for the natural logarithm and "h" represents the number of meters above sea level.
Source These two formulas came from page 71 of Solar Radiation and Daylight Models 2nd edition. The authors
are Tariq Muneer, C. Gueymard, and H. Kambezidis. This edition was published in 2004 by
Elsevier Ltd. ISBN: 0 7506 5974 2
3. The relationship between air mass and insolation was found using pveducation.org's page on air mass. The formulas
used on that page are:
IG = 1.1*ID and ID = 1.353*(0.7)(AM.678) where IG stands for the total global
radiation (this is the number I used in the table) and ID stands for the
intensity of the direct (as opposed to diffuse) light.
The pveducation.org page is http://www.pveducation.org/
Their air mass page is here:
pved. air mass